Class 10 Trigonometry – Easy Notes, Formulas, Examples & MCQs (2025 Update)

Key Learning Outcomes

By the end of this lesson, readers will:

• Introduction to Trigonometry
• Trigonometric Ratios
• Reciprocal Trigonometric Ratios
• Trigonometric Ratios of Standard Angles
• Trigonometric Identities
• Applications of Trigonometry

Introduction

Trigonometry is a branch of mathematics that deals with the relationships between the angles and sides of a right-angled triangle.

Trigonometry ka matlab hota hai “Triangle Measurement” – yani tribhuj ke kon aur sides ke beech ka relation samajhna.

What is Trigonometry?

The word Trigonometry comes from Greek words:

• ‘Tri’ means three
• ‘Gon’ means angles
• ‘Metron’ means measurement

So, Trigonometry = Measurement of triangles.

Trigonometry ka matlab hota hai triangle ke angles aur sides ka connection samajhna aur unhe measure karna — especially jab triangle ka ek angle 90° ho.

Where is Trigonometry Used?

Trigonometry is not just a school subject — it’s used in real life and various professions:

Uses of Trigonometry	Example
Construction	To measure the distance between stars and planets
Astronomy	To measure distance between stars and planets
Navigation	To find direction and position
Engineering	Bridge and road designs
Architecture	Making blueprints and models
Physics	Motion, waves, and light calculations

Tip: Jab kisi tower ki height ya tree ka distance ground se pata karna ho, wahan trigonometry ka use hota hai.

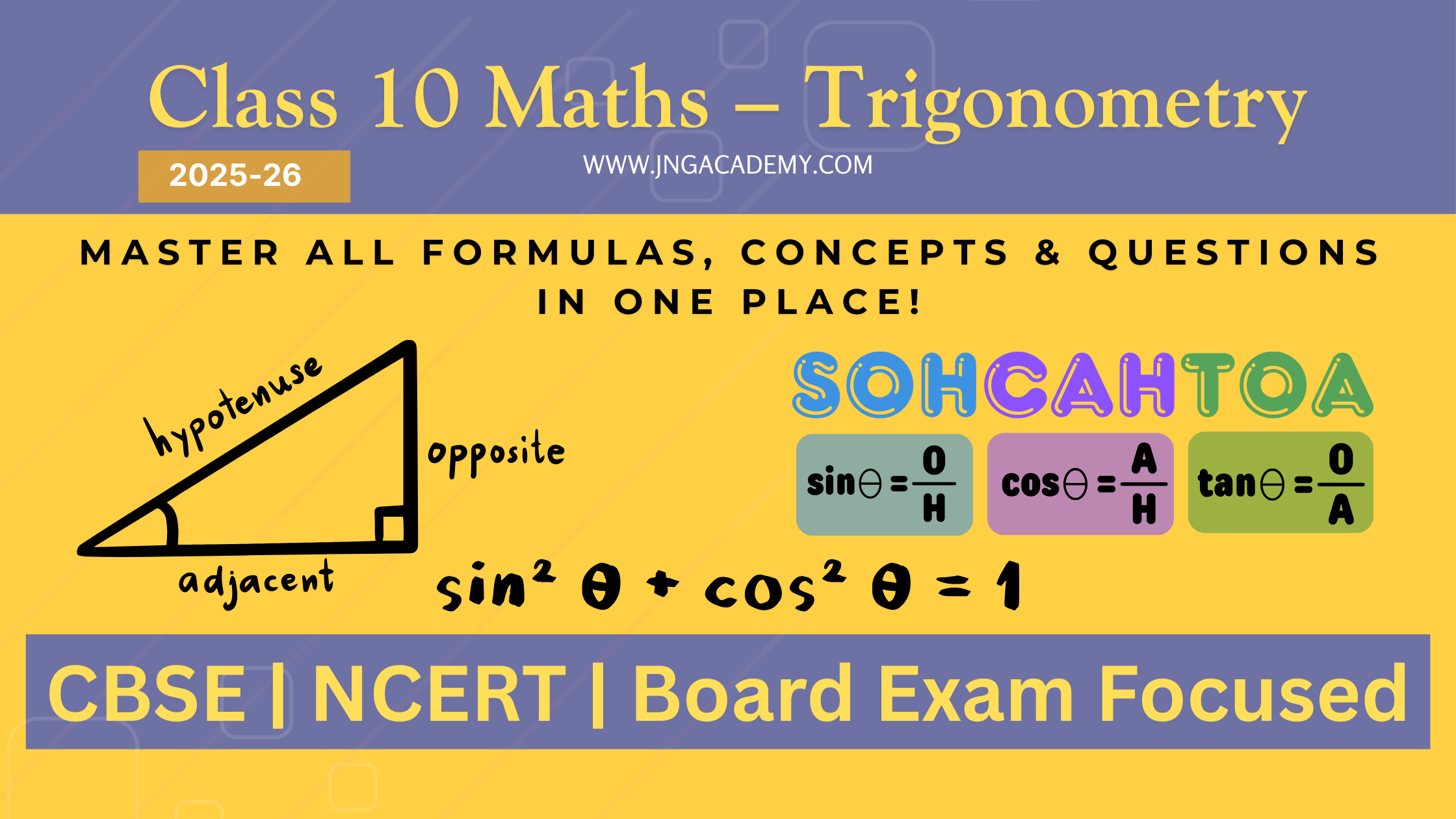
Trigonometric Ratios

      .C
    / |
   /  |
  /   | (Perpendicular=BC)
 /    |
A__B
   (Base=AB)
(Hypotenuse = AC)

Trigonometric Ratios

There are six main trigonometric ratios:

Ratio	Formula (in Right Triangle)
sin θ	Perpendicular / Hypotenuse
cos θ	Base / Hypotenuse
tan θ	Perpendicular / Base
cosec θ	Hypotenuse / Perpendicular
sec θ	Hypotenuse / Base
cot θ	Base / Perpendicular

Example: Agar triangle me Perpendicular = 3, Base = 4, Hypotenuse = 5 hai, to
sin θ = 3/5, cos θ = 4/5, tan θ = 3/4

Note:
tan θ = sin θ / cos θ
cot θ = 1 / tan θ
sec θ = 1 / cos θ
cosec θ = 1 / sin θ

Easy Way to Remember: SOH-CAH-TOA

This is a simple way to remember formulas:

• Sin θ = Opposite / Hypotenuse
• Cos θ = Adjacent / Hypotenuse
• Tan θ = Opposite / Adjacent

Standard Angle Values Table

Angle (θ)	sin θ	cos θ	tan θ	cosec θ	sec θ	cot θ
0°	0	1	0	∞	1	∞
30°	1/2	√3/2	1/√3	2	2/√3	√3
45°	1/√2	1/√2	1	√2	√2	1
60°	√3/2	1/2	√3	2/√3	2	1/√3
90°	1	0	∞	1	∞	0

Ye table yaad karna bohot important hai board exam ke liye!

sin values → 0, 1/2, 1/√2, √3/2, 1
cos values → reverse of sin values
tan = sin / cos

Trigonometric Identities

Most Important Identity:

sin²θ + cos²θ = 1

1 + tan²θ = sec²θ

1 + cot²θ = cosec²θ

Iska matlab agar kisi bhi angle ka sin aur cos ka square add karo to result hamesha 1 hoga.

Complementary Angles in Trigonometry

If two angles add up to 90°, they are complementary.
In such cases:

Identity	Meaning
sin (90° – θ) = cos θ	sine becomes cosine
cos (90° – θ) = sin θ	cosine becomes sine
tan (90° – θ) = cot θ	tangent becomes cotangent
cot (90° – θ) = tan θ	cotangent becomes tangent
sec (90° – θ) = cosec θ	secant becomes cosecant
cosec (90° – θ) = sec θ	cosecant becomes secant

Tip: 90° me se angle ghatao, to ratio complementary ban jaata hai (sin → cos, tan → cot, etc.)

Applications of Trigonometry

Trigonometry is used to calculate heights and distances.

This section includes real-life problems using trigonometry:

• Angle of Elevation: Line of sight above the horizontal (upar dekhna)
• Angle of Depression: Line of sight below the horizontal (neeche dekhna)

Important: Always make a diagram before solving.

Tip:
Jab aap kisi tower ya building ko upar dekhte ho — wo angle of elevation hota hai.
Jab aap upar se neeche kisi object ko dekhte ho — angle of depression.

Real Life Example

Problem: You are standing 50 meters away from a tree. The angle of elevation to the top of the tree is 30°.
Question: What is the height of the tree?

Solution using Trigonometry: Let height = h
tan 30° = h / 50
⇒ 1/√3 = h / 50
⇒ h = 50 / √3 ≈ 28.87 meters

Conclusion: Aap bina tree ke paas jaaye uski height nikal sakte ho — sirf angle aur distance ke basis par!

Solved Example

Q. A boy is standing 20 m away from a pole. The angle of elevation to the top of the pole is 30°. Find the height of the pole.

Ans:
Use formula: tan θ = height / distance
tan 30° = h / 20
1/√3 = h / 20
h = 20 / √3 ≈ 11.55 m

Previous Year Questions

1. Very Short Answer Type Questions (1 Mark)

Q1. Find the value of:
sin 60° × cos 30° + sin 30° × cos 60°
(CBSE 2023)

Solution:
sin 60° = √3/2, cos 30° = √3/2
sin 30° = 1/2, cos 60° = 1/2
= (√3/2 × √3/2) + (1/2 × 1/2)
= (3/4) + (1/4) = 1

Q2. Find the value of:
tan² 45° + sin² 30° – cos² 60°
(CBSE 2022)

Solution:
tan 45° = 1 → tan² 45° = 1
sin 30° = 1/2 → sin² 30° = 1/4
cos 60° = 1/2 → cos² 60° = 1/4
= 1 + 1/4 – 1/4 = 1

2. Short Answer Type Questions (2 Marks)

Q3. Prove that:
(1 + tan² A) = sec² A
(CBSE 2021)

Solution:
This is a standard trigonometric identity.
LHS = 1 + tan² A = 1 + (sin² A / cos² A)
= (cos² A + sin² A) / cos² A = 1 / cos² A
= sec² A = RHS proved

Q4. If sin A = 3/5, and A is acute, find cos A and tan A.
(CBSE 2020)

Solution:
sin A = Opposite/Hypotenuse = 3/5
⇒ Using Pythagoras: Base² = 5² – 3² = 25 – 9 = 16 ⇒ Base = 4
cos A = Base / Hypotenuse = 4/5
tan A = Opposite / Base = 3/4
Answer: cos A = 4/5, tan A = 3/4

3. Application-Based Questions (3 Marks)

Q5. A man is standing 40 m away from a building. The angle of elevation to the top is 30°. Find the height of the building.
(CBSE 2023)

Solution:
tan θ = height / distance
tan 30° = h / 40
⇒ 1/√3 = h / 40 ⇒ h = 40 / √3 = 13.33√3 m ≈ 23.09 m

Q6. If cos A = 12/13, find all other trigonometric ratios of angle A.
(CBSE 2019)

Solution:
cos A = Base / Hypotenuse = 12/13
Using Pythagoras:
Perpendicular² = 13² – 12² = 169 – 144 = 25 ⇒ P = 5

• sin A = P/H = 5/13
• tan A = P/B = 5/12
• sec A = 13/12
• cosec A = 13/5
• cot A = B/P = 12/5

4. Long Answer Type Questions (4–5 Marks)

Q7. A kite is flying at a height of 30 m. The string attached to the kite makes an angle of 60° with the ground. Find the length of the string.
(CBSE 2020)

Solution:
sin θ = Perpendicular / Hypotenuse
sin 60° = 30 / x ⇒ √3/2 = 30 / x ⇒ x = 30 × 2 / √3 = 60 / √3 = 20√3 ≈ 34.64 m

Q8. From the top of a tower 50 m high, the angle of depression to a car is 30°. Find the distance of the car from the base of the tower.
(CBSE 2021)

Solution:
tan θ = Opposite / Adjacent ⇒ tan 30° = 50 / x
1/√3 = 50 / x ⇒ x = 50√3 ≈ 86.60 m

Remembering Points

1. Trigonometry deals only with right-angled triangles and the relationships between their sides and angles.

2. There are six trigonometric ratios:

• sin, cos, tan, cosec, sec, and cot.

3. Key formulas to remember:

sin θ = Perpendicular / Hypotenuse  
cos θ = Base / Hypotenuse  
tan θ = Perpendicular / Base  
cosec θ = Hypotenuse / Perpendicular  
sec θ = Hypotenuse / Base  
cot θ = Base / Perpendicular  

4. Identity to remember:

• sin² θ + cos² θ = 1 (Always true)

5. Standard angles and their values should be memorized:

• sin, cos, tan for 0°, 30°, 45°, 60°, 90°

6. tan θ = sin θ / cos θ and cot θ = cos θ / sin θ

7. tan 90° and cot 0° are not defined

8. Complementary angles identity:

• sin(90° – θ) = cos θ, tan(90° – θ) = cot θ, etc.

9. In real-world applications (height & distance):

• Use tan θ if both perpendicular and base are involved.
• Use sin θ or cos θ if hypotenuse is involved.

10. Always draw a clear triangle diagram when solving height and distance problems.

“Trigonometry ka game hai triangle ke teen sides aur ek angle ke beech relation ka. Formulas yaad rakho aur triangle properly label karo – questions apne aap easy lagne lagenge!”

Interesting Facts About Trigonometry

💡 Ancient Science:
Trigonometry is one of the oldest branches of mathematics! It was first developed by ancient astronomers to study stars and planets, especially by Indian, Greek, and Arab scholars.

💡 Origin of the Word:
The word “Trigonometry” comes from the Greek words:

• Tri = three
• Gon = angles
• Metron = measure
  So, it literally means “measurement of three angles.”

💡 Used to Build Pyramids!
Ancient Egyptians are believed to have used basic trigonometry to construct the pyramids, long before modern tools were invented.

💡 Hidden in Everyday Life:
Trigonometry is used in places you wouldn’t expect – like mobile phone signals, music, games, architecture, satellites, and even in your favorite animated movies!

💡 It Works Even Without a Triangle!
Though it’s based on triangles, trigonometric functions are used in circular motion too. In fact, it helps describe wave patterns like sound waves, light waves, and even heartbeat signals (ECG).

💡 90-Degree Magic:
The whole chapter works around right-angled triangles. If there’s no 90°, it’s not pure trigonometry—it’s something more advanced like spherical trigonometry used by NASA!

💡 Used in Google Maps 📍
Trigonometry helps in finding shortest distances on curved surfaces like Earth! That’s how Google Maps calculates routes so fast.

💡 Angle of Elevation & Depression:
This is more than a math problem – it’s used by pilots, engineers, surveyors, and military to calculate accurate measurements from a distance.

💡 Memory Magic with Tables:
The trigonometric ratio table (0°, 30°, 45°, 60°, 90°) is a magical tool. Once you learn it, many questions feel like a shortcut.

💡 Essential for Engineering 🔧
Trigonometry is the foundation for almost every engineering field – civil, mechanical, aerospace, electrical, and even computer graphics.

Quiz:

Coming Soon…

Supplementary Materials:

Provide downloadable materials for learners to review:

• – PDF Guide: “Coming Soon”
• – Cheat Sheet: “Coming Soon”
• – Video Source: “JNG ACADEMY“
• – Articles: “Blog Page“

FAQs:

More Links:

Paid Earning Courses:

• WordPress for Beginners
• WordPress Freelancing Mastery Course
• Blogging for Beginners